Table of polyhedron dihedral angles
The dihedral angles for the edge-transitive polyhedra are:
| Picture | Name | Schläfli symbol |
Vertex/Face configuration |
exact dihedral angle (radians) |
approximate dihedral angle (degrees) |
|---|---|---|---|---|---|
| Platonic solids (regular convex) | |||||
| Tetrahedron | {3,3} | (3)3 | arccos(1/3) | 70.53° | |
| Hexahedron or Cube | {4,3} | (4)3 | π/2 | 90° | |
| Octahedron | {3,4} | (3)4 | π − arccos(1/3) | 109.47° | |
| Dodecahedron | {5,3} | (5)3 | π − arctan(2) | 116.56° | |
| Icosahedron | {3,5} | (3)5 | π − arccos(√5/3) | 138.19° | |
| Kepler-Poinsot solids (regular nonconvex) | |||||
| Small stellated dodecahedron | {5/2,5} | (5/2)5 | π − arctan(2) | 116.56° | |
| Great dodecahedron | {5,5/2} | (5)5/2 | arctan(2) | 63.435° | |
| Great stellated dodecahedron | {5/2,3} | (5/2)3 | arctan(2) | 63.435° | |
| Great icosahedron | {3,5/2} | (3)5/2 | arcsin(2/3) | 41.810° | |
| Quasiregular solids (Rectified regular) | |||||
| Tetratetrahedron | 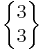 |
(3.3.3.3) | π − arccos(1/3) | 109.47° | |
| Cuboctahedron | 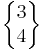 |
(3.4.3.4) | π − arccos(1/sqrt(3)) | 125.264° | |
| Icosidodecahedron |  |
(3.5.3.5) | 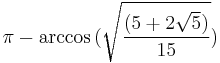 |
142.623° | |
| Dodecadodecahedron | 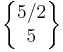 |
(5.5/2.5.5/2) | |||
| Great icosidodecahedron | 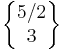 |
(3.5/2.3.5/2) | |||
| Quasiregular dual solids | |||||
| Dual of tetratetrahedron | - | V(3.3.3.3) | π − π/2 | 90° | |
| Rhombic dodecahedron (Dual of cuboctahedron) |
- | V(3.4.3.4) | π − π/3 | 120° | |
| Rhombic triacontahedron (Dual of icosidodecahedron) |
- | V(3.5.3.5) | π − π/5 | 144° | |
References
- Coxeter, Regular Polytopes (1963), Macmillian Company
- Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8 (Table I: Regular Polytopes, (i) The nine regular polyhedra {p,q} in ordinary space)
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-7 to 3-9)